Quantum computers and Qubits

Never give up, turn your weakness into power. Science has no limits and we should never quit trying because there are always possibilities and solutions.

We talked about how computing science has almost achieved its dead-end due to the phenomenon of quantum tunneling that prevents the manufacturing of smaller computer devices.

Don't worry, every time you fall into trouble just call our loved superhero "Quantum mechanics".     I

What does quantum mechanics have to do with computers?

Quantum computers are very similar to classical computers but so much faster and can execute an enormous quantity of information.

The major idea of a quantum computer is depending on eigenvalues superposition. We talked previously about spin which can be all over the place when we're not measuring it but when we do the positions collapses into one single eigenstate either up or down. The image can be clearer if we compared this to tossing a coin in the air because when we do the coin spins very fast in the air and appears like a sphere which is a revolution of a circle around its diameter axis and while it remains in the air all the possible positions are there: vertical, horizontal or deviated by a certain angle. This superposition of states continue until the coin collapses on the ground occupying one of the 2 possible eigenstates: up or down which are respectively attributed to the two bits eigenvalues 1 or 0.

That's why a spin can be assimilated to a bit. 

What I am trying to say is that we need anything that has two possibilities to make it a bit, true or false, cat or dog, tall or short....  Even photons can be used as bits due to their double polarization and all we have to do is passing them through a prism and observe the direction of polarization either horizontal or vertical and attribute 1 or 0 to each one of them.

Is that all? What makes quantum bits special?

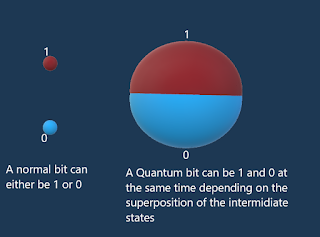
I already answered that. Quantum bits or better known as Qubits have a very interesting and characteristic feature that classical bits doesn't possess which is the ability to occupy two possibilities at the same time.

If a normal bit can be either 1 or 0, a Qubit can be a superposition of these two eigenvalues and to better understand this concept let's consider a very simple example:

what are the possible arrangements of 2 bits?

00

01

10

11

A normal computer which uses classical bits will need to execute 4 operations to find all these solutions by fixing the zero first then complete once by 0 and the other by 1 and vice versa.

Meanwhile, Qubits are more practical since they contain the two values at once, all we need is two operations where the first Qubit takes the value 0 then the other Qubit alternates between 1 and 0 so we get the 00 and 10 possibilities. In the second case, all we need to do is forcing the same first Qubit to take the opposite eigenvalue of 1 and that gives us the rest of the possibilities 10 and 11.

In general, The number of operations Qubits can execute at the same time is 2ⁿ where 2 represents the two possible states that Qubit can occupy and n is the number of Qubits in the computer. 

If we consider a computer using 5 Qubits, the possible simultaneous operations are 2⁵ = 32 while a normal bit computer needs to execute each operation separately.

This number grows up exponentially with every Qubit we add, Imagine a computer with 500 hundred Qubits, that's 2⁵⁰⁰  possible operations !! That's more data than the number of atoms in the observable universe.

This allows us to execute calculations that can demands years for a normal computer!

But something's still confusing, we all know that measuring states of a spin doesn't control the outcome, we just obtain a random up or down positions and eventually, the processed data will be also random. The key to salvation remains in the analogs of logic gates which are known as Quantum gates.

These gates modify the states of the measured spin. For example, a measurement gate measures the initial random spins. On the other hand, a swap gate switches two qubit's values so 01 becomes 10...

There are a lot of possibilities of inverting, adding... that require complex numbers and matrixes ...

To conclude, we can start from every possible Qubits measurement and apply the necessary modifications to obtain the result that we want.

That's not all, we can't talk about computing and data transmission without talking about security.

How secure is a quantum information?

Well actually, It is so secure that is almost unhackable!! 

Imagine you're a hacker who decides to hack a quantum computer with quantum data running all over the place through an optic fiber in the shape of polarised photons.

Hackers need the set of initial data to apply modifications and changes and create a loophole within the targeted system so obviously the first thing you need to do is gathering a sample of that data and here is the tricky thing, If you manage to access the data it's like conducting an active measurement, the superposition of Qubits changes because the measurement is different it's like installing a measurement gate. Copy the Qubits is no solution either.

 This is the strength of quantum mechanics, you can't expect to find a diffracted electron at the same spot unless you stayed for so long. What is the probability that you measure the same data at the same state when it was initially executed? It's like tossing thousands of coins in the air two consecutive times and expect the second time to be the same as the first, in numbers and orders.

Bad news for hackers, Quit what you're doing and go milk some cows.